| |
Page 1 - Introduction and Backgrounds |
|
Page 2 - Polygons and surfaces |
|
Page 3 - Nest, Polyhedra, SF's, Units |
|
Page 4 - The 34 different nests |
|
Page 5 - Animation of the 34 nests, grouped per kind of polygon: |
|
Page 6 - How can a nest be constructed in a given (closed) polygon: |
|
Page 7 - From nest to polyhedron to spacefiller: example SF #1. |
|
Page 8 - From Spacefiller to Unit to 3D-Tiling: |
|
Page 9 - Showing the dual network of the last animation: |
|
Page 10 - 6 Different variations of this Spacefiller #1: |
|
Page 11 - A more complicated example: Polyhedra of Spacefiller #41: |
|
Page 12 - From Polyhedra to SF for #41: |
|
Page 13 - Make a 3D-tiling: One, two, four and eight SF blocks, copied in 3 directions along rhombohedron raster. |
|
Page 14 - One more example of a 3D tiling: SF #14 |
|
Page 15 - The 54 different Polyhedra |
|
Page 16 - The 42 different Spacefillers |
|
Page 17 - All the 16 SF’s made by one kind of nest. |
|
Page 18 - SF animations of SF01-SF14 |
|
Page 19 - SF animations of SF15-SF28 |
|
Page 20 - SF animations of SF29-SF42 |
|
Page 21 - Z-Patterns of nests |
|
Page 22 - Some statistics and the structure-graph |
|
Page 23 - 3 Tables with details of: Spacefillers, Polyhedra and Nests |
|
Page 24 - Projections of Dual Nets (SF# 1,28,41) |
|
Page 25 - Credits and Acknowledgements |
|
| |
- Collection of Spacefillers (SF’s)
- Based on this book by Peter Pearce (1978), chapter 8.
- Spidronisation of these SF’s gives some new insights into 3D tilings.
- More details can be found in our paper published in the Bridges 2009 Conference Proceedings, or click here.
|
 |
|
 |
| Faces of Saddle Polyhedra can be defined with Skew Polygons. |
 |
 |
| These can be filled with a minimal surface like a soap film, as Pearce did. |
 |
 |
| Or with Spidronnests. This introduces an orientation: CW or CCW (clockwise / counter-clockwise) |
 |
 |
|
 |
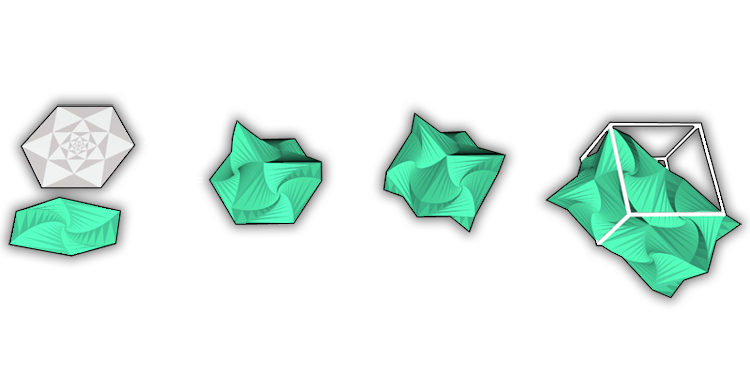 |
In general: Nests are the building blocks of Polyhedra, which can be assembled into SF’s, and to (possibly bigger) periodical Units.
Matching nests in the 3D tiling need to have opposite orientations.
|
|
 |
|
 |
Grey: flat
Blue: regular
Red: mirror 4-gon
Purple: mirror 5-gon
Turquoise: mirror 6-gon
Violet: mirror 8-gon
Green: mirror 12-gon
Yellow: enantiomorphic
|
|
| |
|
|
 |
|
| Method: “scale and rotate”. Nest in general not foldable. |
|
 |
|
| Spidronnest orientations causes doubling compared to Pearce’s SF’s. |
|
 |
|
 |
|
| Cyan is CCW and Blue is CW. Projection along raster gives 2D-tilings. |
|
 |
|
 |
|
 |
|
 |
|
 |
|
 |
|
 |
|
 |
(SF’s 1 2 3 4 / 6 9 11 13 / 14 23 24 27 / 28 30 33 39 ) |
 |
|
 |
|
 |
|
 |
|
 |
|
 |
|
 |
|
 |
|
 |
- Thanks to Peter Pearce, who described in his great book “Structure in Nature is a Strategy for Design” all the original Spacefillers, based on saddle-surfaces.
- Thanks to all other members of the Spidron Team (amongst others: Lajos Szilassi, Rinus Roelofs, Marc Pelletier, Amina Buhler-Allen), for all their inspiring earlier work, including four earlier designs of Spidronised Spacefillers.
(SF’s 2 , 3 , 4 and 5, all based on only regular skew polygons)
- Thanks to András Fodor, who made this presentation.
- Apart from the abovementioned contributions, all work presented here was made by:
Walt van Ballegooijen, Paul Gailiunas and Dániel Erdély, 2009.
- For more information about Spidrons, see the main website:
www.spidron.hu.
|
|
 |




